Why John Nash Matters, Rock-Paper Scissors, Pitch Sequencing and Hitting

This is why statistics if fun....the more you know, the more you have to learn.

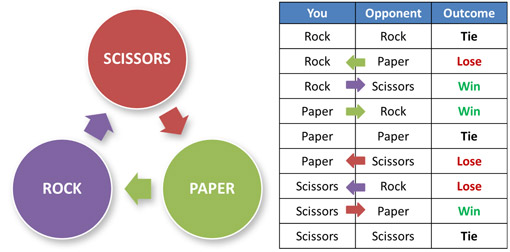
from fivethirtyeight.com

http://fivethirtyeight.com/datalab/why-john-nash-matters/

Nash's 1951 article "Non-Cooperative Games" refined the definition of an "equilibrium" as a situation in which each player is employing a strategy that is optimal given the strategies of all the other players. For example, in the Prisoner's Dilemma — a game formalized by Nash's thesis adviser Albert W. Tucker — the two suspects betraying each other is an equilibrium, despite the best overall outcome being for both of them to remain silent. Nash's definition would (appropriately) become known as the "Nash Equilibrium" — a term familiar to students in a wide range of academic disciplines.

Given this new definition, Nash was able to prove that a "mixed-strategy" equilibrium exists for virtually any finite game. A "mixed" strategy is one where, instead of choosing a single action, a player chooses a mix of actions with a certain probability for each — for example, the strategy of choosing rock, paper or scissors one-third of the time each in Rock Paper Scissors. It doesn't matter if your opponent knows what strategy you're playing, they can't do anything about it.

One area in which Nash's legacy continues to be especially relevant and fruitful is sports analysis. One of the cleanest examples is the mini-game of penalty kicks in soccer. If a player always kicks the same direction, the goalkeeper can profitably adapt by always diving in that direction. Thus "always kick right" or "always kick left" can't be equilibrium strategies. Similarly, if the goalkeeper always dived in one direction, the player would be able to profitably deviate by kicking in the other. Thus the equilibrium strategy for the kicking player is to "mix it up" (use a mixed strategy) by kicking one way some of the time and the other way some of the time. Presuming the player selects randomly and doesn't telegraph his moves, the goalkeeper can do no better than guessing. Therefore, the goalkeeper's equilibrium response is to also mix it up, diving in each direction often enough to keep the kicker from exploiting his tendencies. This is why you often see wildly inaccurate dives: It's not necessarily because they were faked out, it's just that they picked scissors when the striker picked rock.

Sent from my iPhone

--

http://makenolittleplans.net/a-new-view-on-pitch-sequencing/

A NEW VIEW ON PITCH SEQUENCING

The core of baseball is the duel between pitcher and batter.  The pitcher's job is to throw a baseball past the batter, or otherwise induce the batter to make an out (via a weakly hit ball, for instance).  The batter's job is the opposite: to make solid contact with the ball, transferring enough force to either cause the batted ball to be difficult to field (e.g. a line drive) or impossible to field (a home run).

An interesting aspect of this battle between pitcher and batter is the fact that the batter can, in theory, hit any pitch within the strike zone (at the major league level).  It is straightforward physics to reason that, given enough information, a batter can make good contact with any strike, and indeed, MLB hitters can get hits from even 100+ mph fastballs (albeit rarely).

The fastest pitches still take about four-tenths of a second to go from hand to plate, which is more than enough time to swing.  I would argue that the crucial weapon the pitcher has in his arsenal isn't speed, it's uncertainty.  If a pitcher can cause a batter to adjust their swing mid-cut, or not swing at all, that is a vastly more powerful advantage than the milliseconds that can be chopped off by simply throwing harder.

For the remainder of this post, I'll investigate how pitchers utilize uncertainty in their pitch sequences.  MGL has hypothesized (or perhaps has data to show?) that pitchers optimize their sequencing of pitches such that the identity of the first pitch in a sequence gives no information as to the identity of the next pitch.  That is to say, conditional on the first pitch being, say, a fastball, the batter has no more idea of what the next pitch will be than he did before the first pitch.

 Baseball Prospectus | The Art and Science of Sequencing

				Baseball Prospectus | The Art and Science of Sequencing A first foray into one of baseball's most murky subjects.
				View on www.baseballprospe...	Preview by Yahoo

Pitch sequencing has long lurked as a sort of terra incognita in sabermetric analysis. It's something that all baseball folks agree is important, but it's proved mostly impenetrable to strictly quantitative approaches. There's an intuitive sense that sequencing must be one of the crucial determinants of pitcher success, and although we can seemingly identify a good sequence when we see one, any attempt to apply a universal criterion of good sequencing across all pitches (or pitchers) is much more challenging. The rest of this article will be devoted to applying just such a criterion, and determining whether it is of any practical utility in understanding pitching generally.

There are at least two schools of thought about pitch sequencing. On the one hand, there seems to be an appreciation for sequences that mix up locations, speeds, and breaks in unpredictable ways, on the grounds that those kinds of sequences ought to be the most challenging for a hitter. On the other hand, Mitchel Lichtman (aka MGL) has argued forcefully on the basis of game theory that the ideal sequencing would be something like weighted randomness (weighted, that is, by the quality of each pitch). MGL's argument says that if a pitcher tried too hard to mix things up, for instance by purposefully not throwing two of the same pitch in a row, he would end up tipping the next pitch to the batter, resulting in a powerful disadvantage.

I believe that it's instructive to consider an analogy to rock-paper-scissors. Any player of rock-paper-scissors knows that it's best not to establish any predictable tendencies. Should a player begin to always follow his "rocks" with "papers," it becomes a trivial exercise to counter with "scissors." This line of argument represents the weighted randomness school of MGL. On the other hand, very sophisticated players of rock-paper-scissors can predict patterns in novices or observe them in experts; countering these subtle tendencies may involve nonrandom usage of the various symbols (i.e. rock/paper/scissors). By analogy, this latter strategy would represent the more classical school of sequencing.

It's important to note that these two schools are not necessarily contradictory, even though they appear to be at first glance. A pitcher may be carefully crafting his sequences in a single game or against a certain batter or team—in short, on a small scale. However, these individual sequences may all even out at the scope of a full season, yielding MGL's pattern of randomness. To that point, an important caveat for the following is that I will look at sequencing at the level of a whole season (in order to maximize sample size). But if there are interesting patterns at the scale of individual games or months or at-bats, they'll have to wait for further analysis.

.....

Sequencing in Context
It's tough to imagine Joey Votto at the plate taking the logarithms of the frequencies of four-seam fastballs in order to predict the next pitch. But then again, maybe he doesn't have to; maybe it's the job of some poor scout in the Reds' front office to pick out pitch sequence tendencies, and Votto merely knows which two pitches to look for such that the next pitch is easily predictable. Maybe, too, Votto gets a hunch once in a while, an intuition or a feeling, that directs him to be aware of the possibility of a slider more than another pitch.

That constant struggle to predict the pitcher's next move forces the pitcher to vary his repertoire as much as he is able, given the count and the quality of his pitches. Yet, perhaps because of those constraints, no pitcher approaches the theoretical optimum of perfectly random sequencing. Returning again to the dichotomous schools of sequencing, I think my results provide something for both sides to appreciate. On the one hand, lower mutual information correlates with better pitchers (though causation is much more difficult to pin down). On the other, even when the outcome is favorable to the pitcher, pitchers aggressively structure their sequences. The results are therefore somewhat inconclusive.

There remains much to be investigated. As mentioned above, this view on sequencing ignores one of the most crucial sources of uncertainty in pitching, namely location. Understanding location in the context of entropy is very tricky, but necessary, and I'll look to tackle that in the future. In addition, there's an entire other party in calling pitches to whom I've given little mention: the catcher. Which catchers sequence well, and which poorly? Finally, it would be desirable to connect individual sequences of pitches to good or bad results, looking for situations in which pitchers establish predictable sequences and are then punished. So look upon this article as a first foray into a previously unexplored area of sabermetrics, one in which there appears to be much more to be discovered on account of the fact that there's so little known.

Robert Arthur is an author of Baseball Prospectus.   
Click here to see Robert's other articles. You can contact Robert by Baseball Prospectus | | Contact Us

from Business Insider:

http://www.businessinsider.com/how-to-beat-anyone-at-rock-paper-scissors-2014-5

The question of how to win at Rock-Paper-Scissors has, believe it or not, plagued mathematicians and game theorists for quite some time. While they previously had devised a theoretical answer to the question, a new experiment by Zhijian Wang at Zhejiang University in China that used real players, has revealed an interesting wrinkle to the original theory.

In the experiment, Zhijian noticed that winning players tended  to stick with their winning strategy, while losers tended to switch to the next strategy in the sequence of rock-paper-scissors, following, what he calls, “persistent cyclic flows.”

Here's how it works in practice: Player A and Player B both start by using random strategies. If Player A uses rock and Player B uses paper, Player A loses. In the next round, Player A can assume that Player B will use paper again and should therefore use scissors to win. In the round after that, because Player B lost, Player A can assume that Player B will use the next strategy in the sequence — scissors — and Player A should then use rock, thus winning again. 

If you take the game on a theoretical level, the most mathematically sound way to play Rock-Paper-Scissors is by choosing your strategy at random. Because there are three outcomes — a win, a loss, or a tie — and each strategy has one other strategy that it can beat and one other strategy that can beat it, and we don’t care what strategy we win with, it makes the most sense to pick paper exactly 1/3 of the time, rock 1/3 of the time, and scissors 1/3 of the time. This is called the game's Nash equilibrium. 

While the Nash Equilibrium should be the best strategy in real life, Zhijian found a decidedly different pattern when he and some other researchers recruited 72 students to play the game. They divided the students into 12 groups of six players and had them each play 300 rounds of Rock-Paper-Scissors against each other. Zhijian also added a payout in proportion to the number of victories.

Harrison Jacobs





Losing players choose the next strategy in the sequence.

When Zhijian reviewed the results he found that students chose each strategy close to one third of the time, suggesting the Nash Equilibrium theory. However, when he looked closer, he noticed a more unusual pattern.

The pattern that Zhijian discovered — winners repeating their strategy and losers moving to the next strategy in the sequence — is called a “conditional response” in game theory. The researchers have theorized that the response may be hard-wired into the brain, a question they intend to investigate with further experiments.

For now, Zhijian suggests that exploiting the knowledge that most people use the conditional strategy may result in winning a lot more games of Rock-Paper-Scissors. 

Read more: http://www.businessinsider.com/how-to-beat-anyone-at-rock-paper-scissors-2014-5#ixzz3bGpjEAa3

from NY Times: 
http://www.nytimes.com/interactive/science/rock-paper-scissors.html?_r=0

Rock-Paper-Scissors: You vs. the Computer

Computers mimic human reasoning by building on simple rules and statistical averages. Test your strategy against the computer in this rock-paper-scissors game illustrating basic artificial intelligence. Choose from two different modes: novice, where the computer learns to play from scratch, and veteran, where the computer pits over 200,000 rounds of previous experience against you.

 Note: A truly random game of rock-paper-scissors would result in a statistical tie with each player winning, tying and losing one-third of the time. However, people are not truly random and thus can be studied and analyzed. While this computer won't win all rounds, over time it can exploit a person's tendencies and patterns to gain an advantage over its opponent.

Rock-Paper-Scissors: You vs. the Computer

				Rock-Paper-Scissors: You vs. the Computer Test your strategy against the computer in this rock-paper-scissors game illustrating basic artificial intelligence.
				View on www.nytimes.com	Preview by Yahoo

SMARTER THAN YOU THINK

Rock-Paper-Scissors: You vs. the Computer

http://www.worldrps.com/index.php?option=com_content&task=view&id=256&Itemid=37

How to beat anyone at Rock Paper Scissors

RPS News and Notes

With the 2006 World Rock Paper Scissors Championships coming up in November in Toronto and $10,000.00 on the line (not to mention bragging rights of being able to call yourself "World Champion of RPS"), trying to get some sort of edge on your competition is becoming a focal point for a lot of players.

Little bit of background. Contrary to what you might think RPS is not simply a game of luck or chance. While it is true that from a mathematical perspective the 'optimum' strategy is to play randomly, it still is not a winning strategy for two reasons. First, 'optimum' in this case means you should win, lose and draw an equal number of times (hardly a winning strategy over the long term). Second, Humans, try as they might, are terrible at trying to be random, in fact often humans in trying to approximate randomness become quite predictable. So knowing that there is always something motivating your opponent's actions, there are a couple of tricks and techniques that you can use to tip the balance in your favour.

The secret to winning at RPS

Basically, there are two ways to win at RPS. First is to take one throw away from your opponent options. ie - If you can get your opponent to not play rock, then you can safely go with scissors as it will win against paper and stalemate against itself. Seems impossible right? Not if you know the subtle ways you can manipulate someone. The art is to not let them know you are eliminating one of their options. The second way is...READ MORE

With the 2006 World Rock Paper Scissors Championships coming up in November in Toronto and $10,000.00 on the line (not to mention bragging rights of being able to call yourself "World Champion of RPS"), trying to get some sort of edge on your competition is becoming a focal point for a lot of players.

Little bit of background. Contrary to what you might think RPS is not simply a game of luck or chance. While it is true that from a mathematical perspective the 'optimum' strategy is to play randomly, it still is not a winning strategy for two reasons. First, 'optimum' in this case means you should win, lose and draw an equal number of times (hardly a winning strategy over the long term). Second, Humans, try as they might, are terrible at trying to be random, in fact often humans in trying to approximate randomness become quite predictable. So knowing that there is always something motivating your opponent's actions, there are a couple of tricks and techniques that you can use to tip the balance in your favour.

The secret to winning at RPS

Basically, there are two ways to win at RPS. First is to take one throw away from your opponent options. ie - If you can get your opponent to not play rock, then you can safely go with scissors as it will win against paper and stalemate against itself. Seems impossible right? Not if you know the subtle ways you can manipulate someone. The art is to not let them know you are eliminating one of their options. The second way is to force you opponent into making a predictable move. Obviously, the key is that it has to be done without them realizing that you are manipulating them.

Most of the following techniques use variations on these basic principles. How well it works for you depends upon how well you can subtly manipulate your opponent without them figuring out what you are doing. So, now that  the background is out of the way, let's get into these techniques:

Lead on Banner 1 - Rock is for Rookies

In RPS circles a common mantra is "Rock is for Rookies" because males have a tendency to lead with Rock on their opening throw. It has a lot to do with idea that Rock is perceived as "strong" and forceful", so guys tend to fall back on it. Use this knowledge to take an easy first win by playing Paper. This tactic is best done in pedestrian matches against someone who doesn't play that much and generally won't work in tournament play.

2 - Scissors on First

The second step in the 'Rock is for Rookies' line of thinking is to play scissors as your opening move against a more experienced player. Since you know they won't come out with rock (since it is too obvious), scissors is your obvious safe move to win against paper or stalemate to itself.

3 - The Double Run

When playing with someone who is not experienced at the RPS, look out for double runs or in other words, the same throw twice. When this happens you can safely eliminate that throw and guarantee yourself at worst a stalemate in the next game. So, when you see a two-Scissor run, you know their next move will be Rock or Paper, so Paper is your best move. Why does this work? People hate being predictable and the perceived hallmark of predictability is to come out with the same throw three times in row.

4 - Telegraph Your Throw

Tell your opponent what you are going to throw and then actually throw what you said. Why? As long as you are not playing someone who actually thinks you are bold enough to telegraph your throw and then actually deliver it, you can eliminate the throw that beats the throw you are telegraphing. So, if you announce rock, your opponent won't play paper which means coming out with that scissors will give you at worst a stalemate and at best the win.

5 - Step Ahead Thinking

Don't know what to do for your next throw? Try playing the throw that would have lost to your opponents last throw? Sounds weird  but it works more often than not, why? Inexperienced (or flustered) players will often subconsciously deliver the throw that beat their last one. Therefore, if your opponent played paper, they will very often play Scissors, so you go Rock. This is a good tactic in a stalemate situation or when your opponent lost their last game. It is not as successful after a player has won the last game as they are generally in a more confident state of mind which causes them to be more active in choosing their next throw.

Lead on Banner

6 - Suggest A Throw

When playing against someone who asks you to remind them about the rules, take the opportunity to subtly "suggest a throw" as you explain to them by physically showing them the throw you want them to play. ie  "Paper beats Rock, Rock beats scissors (show scissors), Scissors (show scissors again) beats paper." Believe it or not, when people are not paying attention their subconscious mind will often accept your "suggestion".  A very similar technique is used by magicians to get someone to take a specific card from the deck.

7 - When All Else Fails Go With Paper

Haven't a clue what to throw next? Then go with Paper. Why? Statistically, in competition play, it has been observed that scissors is thrown the least often. Specifically, it gets delivered 29.6% of the time, so it slightly under-indexes against the expected average of 33.33% by 3.73%. Obviously, knowing this only gives you a slight advantage, but in a situation where you just don't know what to do, even a slight edge is better than none at all.

8 - The Rounder's Ploy

This technique falls into more of a 'cheating' category, but if you have no honour and can live with yourself the next day, you can use it to get an edge. The way it works is when you suggest a game with someone, make no mention of the number of rounds you are going to play. Play the first match and if you win, take it is as a win. If you lose, without missing a beat start playing the 'next' round on the assumption that it was a best 2 out of 3. No doubt you will hear protests from your opponent but stay firm and remind them that 'no one plays best of one for a kind of decision that you two are making'. No this devious technique won't guarantee you the win, but it will give you a chance to battle back to even and start again.

About the author: Graham Walker

As a co-author of the Official Rock Paper Scissors Strategy Guide (published by Simon and Schuster) and five-time organizer of the World Rock Paper Scissors Championships, Graham Walker is one of the few people who is actually a credible authority on the strategy and cultural significance on the game of Rock Paper Scissors.